Author: Himanshu Lekharu1*
1Research scholar, Jawaharlal Nehru University, New Delhi
*Corresponding author: himanshu.lekharu@gmail.com
Abstract
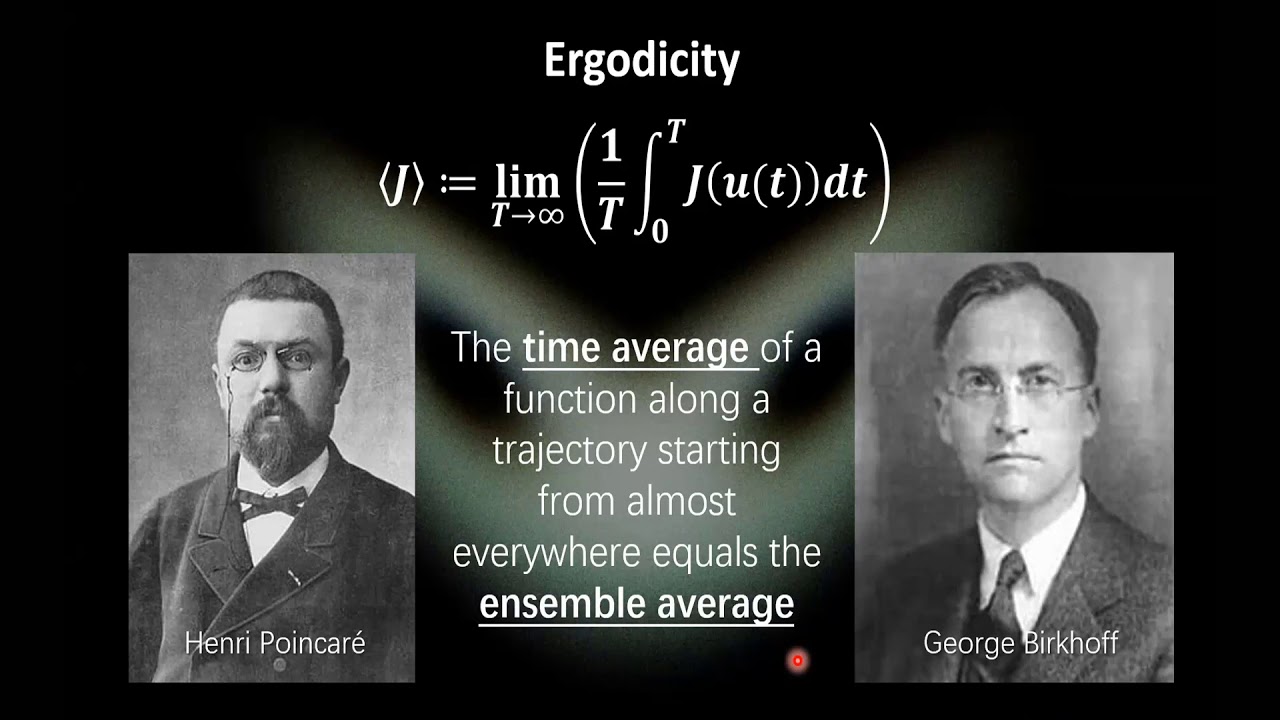
Ergodic theory, a profound mathematical field, delves into the long-term behavior of dynamic systems. This concise overview begins by introducing fundamental measure theory concepts, such as measure spaces and measurable functions. It then explores measure-preserving transformations with examples like Poincar ´e recurrence and introduces the crucial concept of ergodicity, exemplified by irrational rotations. The article culminates in an examination of ergodic theorems, prominently featuring Birkhoff’s ergodic theorem, which elucidates the long-term statistical properties of evolving systems. This exploration provides valuable insights into the captivating world of ergodic theory, with broad applications across diverse domains
Keywords
Poincar ´e Recurrence, Ergodicity, Birkhoff’s Ergodic Theorem